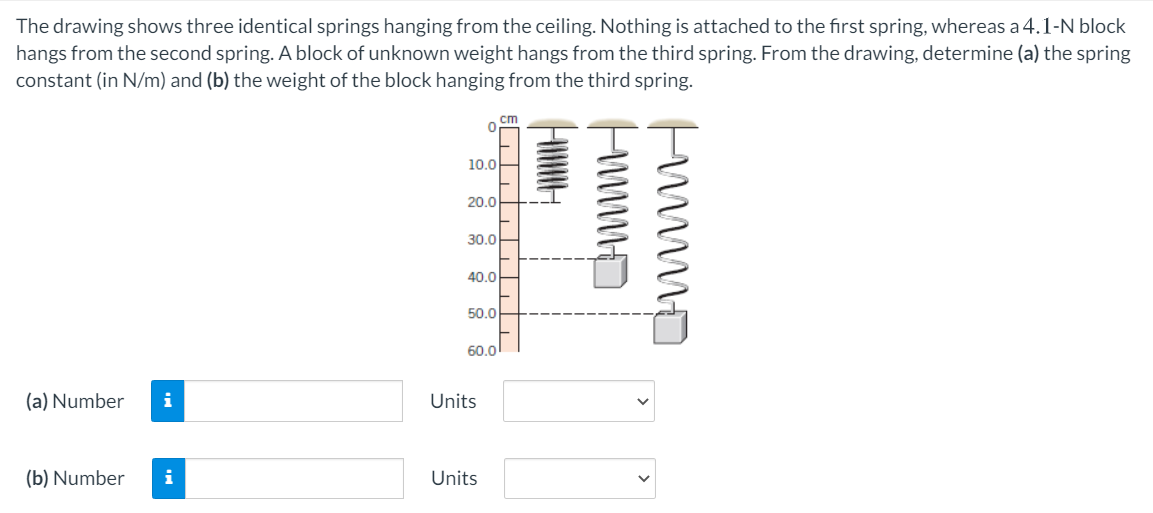
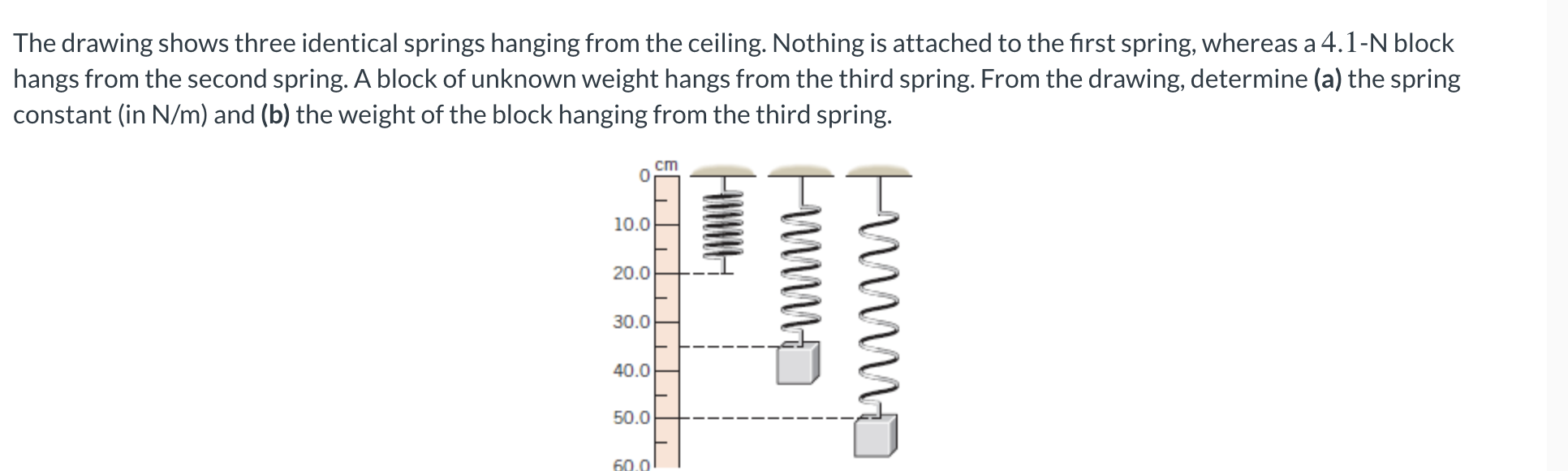
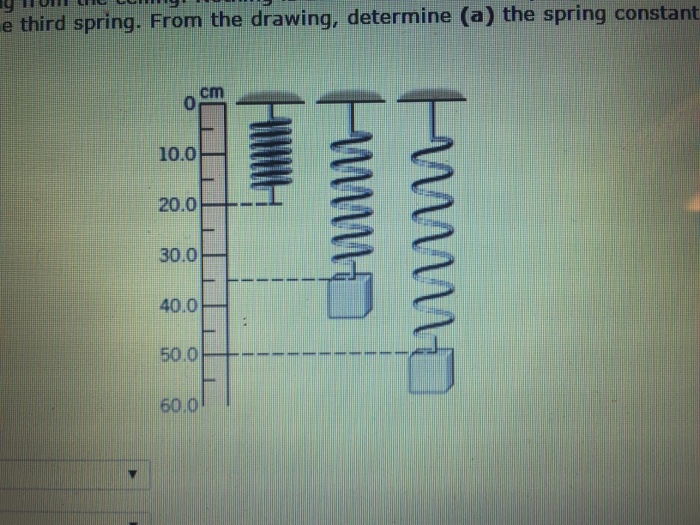
The Drawing Shows Three Identical Springs Hanging From The Ceiling, The drawing shows three identical springs hanging from the ceiling.
The Drawing Shows Three Identical Springs Hanging From The Ceiling - Web the drawing shows three identical springs hanging from the ceiling. The drawing shows three identical springs hanging from the ceiling. Web the drawing shows three identical springs hanging from the ceiling. We can use hooke's law to find the spring constant: There are three identical springs in our house. This one has the weight of it. Web the third spring has a block of unknown weight hanging from it. Nothing is attached to the first spring, whereas a 4. There is nothing hanging on this one. Web the drawing shows three identical springs hanging from the ceiling. Web the drawing shows three identical springs hanging from the ceiling. There is nothing left on this one. Web the drawing shows three identical springs hanging from the ceiling. Web 3 identical springs hanging from ceiling. Nothing is attached to the first spring, whereas a 4.5 n block hangs from the second spring. Web the drawing shows three identical springs hanging from the ceiling. Nothing is attached to the first spring, whereas a 4. Nothing is attached to the first spring, whereas a 4.5 n block hangs from the second spring. The drawing shows three identical springs hanging from the ceiling. There is nothing hanging on this one. Web the drawing shows three identical springs hanging from the ceiling. Web the drawing shows three identical springs hanging from the ceiling. Spring 1 has a constant of k = 4pi^2 (0.7143)/1, spring 2. Nothing is attached to the first spring, whereas a 4.5 n block hangs from the second spring. Web the drawing shows three identical springs hanging from. Web the drawing shows three identical springs hanging from the ceiling: From the drawing, we can determine the weight of the third block by comparing it to the weight of the second block. The spring is then cut into two identical springs of 50. The 4.5 is what this one has. Spring 1 has a constant of k = 4pi^2. The spring is then cut into two identical springs of 50. Web the drawing shows three identical springs hanging from the ceiling. Web the third spring has a block of unknown weight hanging from it. Web the drawing shows three identical springs hanging from the ceiling. We can use hooke's law to find the spring constant: Web the third spring has a block of unknown weight hanging from it. The drawing shows three identical springs hanging from the ceiling. The 4.5 is the one this one has. Web the drawing shows three identical springs hanging from the ceiling. Web the drawing shows three identical springs hanging from the ceiling: Web the drawing shows three identical springs hanging from the ceiling. Web the drawing shows three identical springs hanging from the ceiling. Web the drawing shows three identical springs hanging from the ceiling. Web the drawing shows three identical springs hanging from the ceiling. Web the drawing shows three identical springs hanging from the ceiling. Web the drawing shows three identical springs hanging from the ceiling. The drawing shows three identical springs hanging from the ceiling. There is nothing left on this one. Web 3 identical springs hanging from ceiling. Web the drawing shows three identical springs hanging from the ceiling. We have a spring which has a relaxed length of 20 centimeters, and we're going to convert it to meters by dividing it by 100 pit, and we'll get 0.2 meters when. Web the drawing shows three identical springs hanging from the ceiling. Web the drawing shows three identical springs hanging from the ceiling. The weight is on this one.. Web the drawing shows three identical springs hanging from the ceiling. The 4.5 is the one this one has. Nothing is attached to the first spring, whereas a 4.5 n block hangs from the second spring. The weight is on this one. Nothing attached to the first spring. The spring is then cut into two identical springs of 50. Web the drawing shows three identical springs hanging from the ceiling. Nothing is attached to the first spring, whereas a 4. From the drawing, we can determine the weight of the third block by comparing it to the weight of the second block. The drawing shows three identical springs hanging from the ceiling. Web the drawing shows three identical springs hanging from the ceiling. Web the drawing shows three identical springs hanging from the ceiling. Web the drawing shows three identical springs hanging from the ceiling. We have a spring which has a relaxed length of 20 centimeters, and we're going to convert it to meters by dividing it by 100 pit, and we'll get 0.2 meters when. There is nothing hanging on this one. Spring 1 has a constant of k = 4pi^2 (0.7143)/1, spring 2. Web the drawing shows three identical springs hanging from the ceiling. We have the same springs. Web the drawing shows three identical springs hanging from the ceiling. Web the drawing shows three identical springs hanging from the ceiling: Web the drawing shows three identical springs hanging from the ceiling.Solved The drawing shows three identical springs hanging

Solved The drawing shows three identical springs hanging
Solved The drawing shows three identical springs hanging
Solved The drawing shows three identical springs hanging
Solved The drawing shows three identical springs hanging
SOLVED The drawing shows three identical springs hanging from the
Solved The drawing shows three identical springs hanging
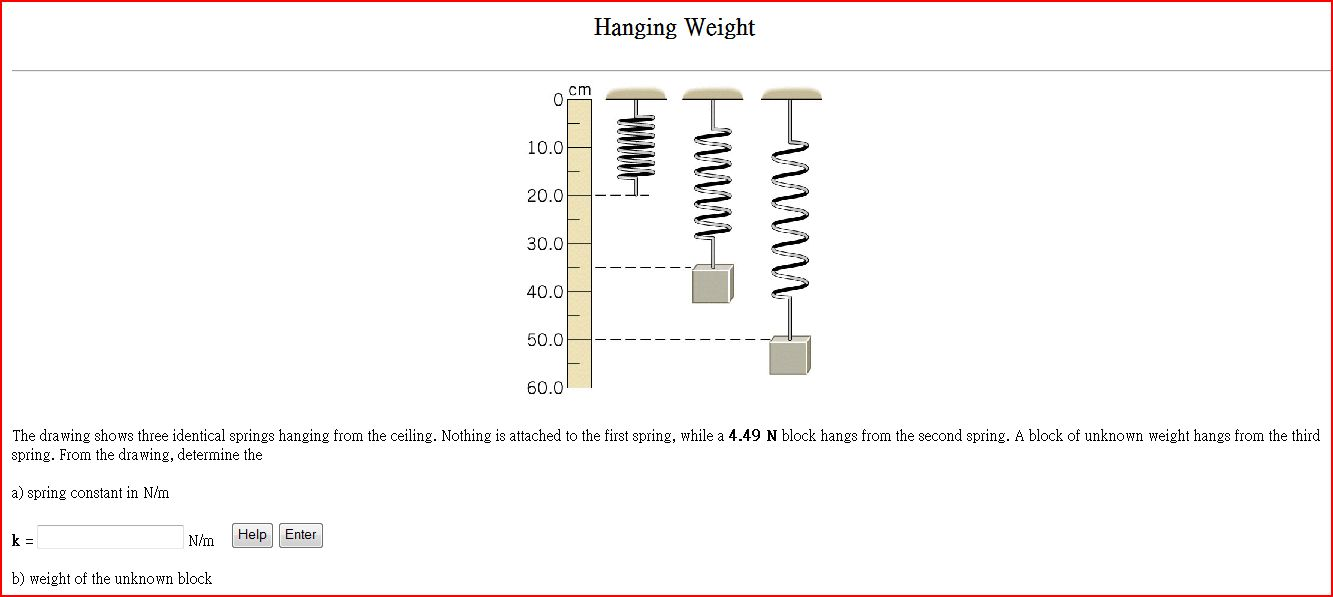
Solved The drawing shows three identical springs hanging
Solved The drawing shows three identical springs hanging
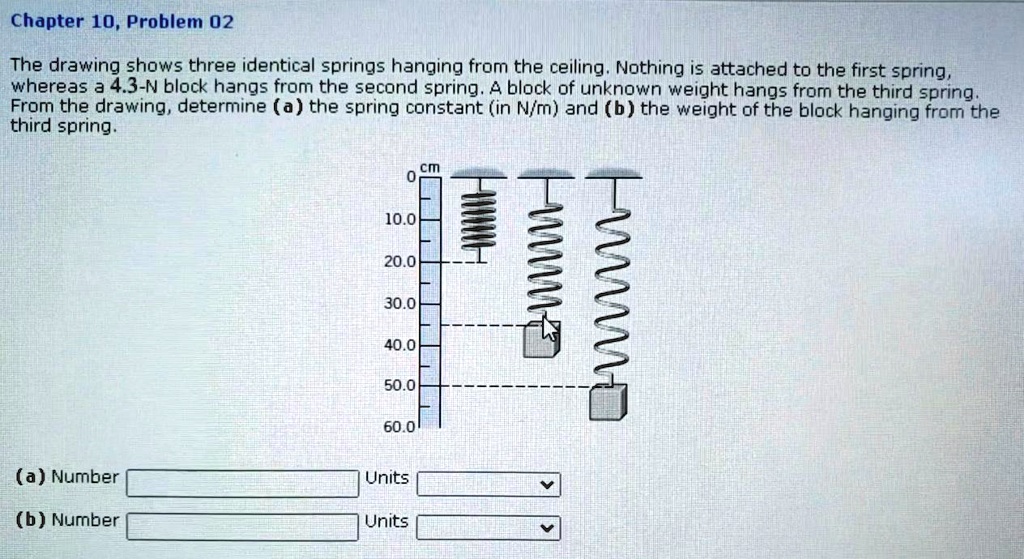
SOLVED Chapter 10, Problem 02 The drawing shows three identical
Web The Third Spring Has A Block Of Unknown Weight Hanging From It.
The 4.5 Is What This One Has.
Web The Drawing Shows Three Identical Springs Hanging From The Ceiling.
The Drawing Shows Three Identical Springs Hanging From The Ceiling.
Related Post: